Seperative Work Units (SWUs) are a measurement of the effort required to seperate isotopes of uranium for use in nuclear power stations or nuclear weapons.
The maths behind the calculation of SWUs is quite complicated (Kirk Sorenson has written a great article about calculating SWUs) but what is interesting is to compare the effort required in various situations.
Examples
Little Boy, the sixteen kiloton nuclear weapon that was dropped on Hiroshima during World War II contained fifty kilograms of uranium enriched to 88% and a further fourteen kilograms enriched to 50%. This would require 10800 SWUs (9350 + 1450).
Aside from its work enriching uranium to 5% for use in the Bushehr nuclear power station, Iran has also enriched 98 kg of uranium to 20% [source], requiring 3740 SWUs. To further enrich this fuel, to produce 20 kg of highly enriched uranium – enough for a nuclear weapon – would require a further 370 SWUs.
Data about nuclear-powered submarines is hard to come by, but unclassified sources state that Ohio Class SSBNs of the US Navy are powered by General Electric S8G nuclear reactors using fuel that has been enriched to 97.3%, probably with an initial fuel load of around 400 kg. To produce 400 kg of fuel enriched to 97.3% would require 83700 SWUs.
Sizewell B is the UK’s newest nuclear power station and produces about two gigawatts of electricity (about seventeen billion kWh per year). It uses about thirty tonnes of uranium enriched to about 3.5% per year, which would require 129000 SWUs.
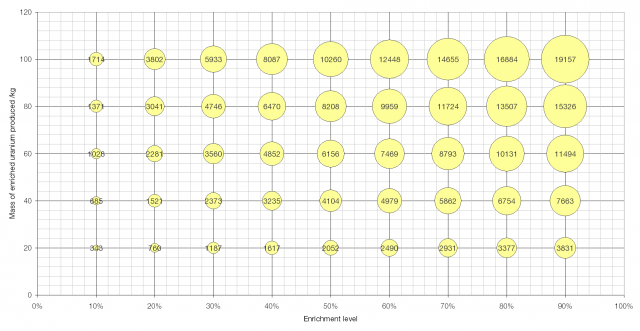
A graph showing the effort required to produce a given amount of enriched uranium to a given level. The area of the bubbles is proportional to the number of SWUs required. Click to enlarge.
It’s worth looking in these cases at the amount of initial uranium required. The greater the desired enrichment level, the greater the initial feed required to yield a given mass of enriched uranium is. In the case of Little Boy, to produce 64 kg of uranium enriched to around 80% would have required more than 12 tonnes (12 096 kg) of initial uranium (and a much larger amount of uranium ore, depending on the grade of ore*). This would result in 12 032 kg of waste depleted uranium, good only for use as ballast, shielding or armor-piercing projectiles. The amount of effort required (the number of SWUs) to enrich this depleted uranium to a usable level would be far too great for proliferation to be a problem.
By far the predominant current method of isotope separation is the use of gas centrifuges, at a cost of around $100 per SWU†; thus the cost of the enrichment required to run Sizewell B for a year would be about $13 million. A newer method, laser enrichment, promises to cut this cost to around $30/SWU, which would bring down the cost of running Sizewell B to only $3.9 million. Unfortunately this would also make enrichment for more nefarious uses cheaper.
SWU calculations depend on the amount of uranium left behind in the “tailings” of the enrichment process. For the purposes of all the figures above this is assumed to be 0.3%. If uranium were to become scarce then this percentage would obviously decrease.
* The highest grade ore in the world comes from the Athabasca Basin in Canda, with a grade of 18%. To yield one kilogram of uranium from Athabasca would require 5.56 kilograms of ore.
† The figures for cost per SWU come from Sharon Weinberger, “Laser plant offers cheap way to make nuclear fuel”, Nature 487: 16-17. DOI: 10.1038/487016a.